

When the marginal product is increasing, the total product increases at an increasing rate. What factors would cause this? As more workers are added, they are able to divide the respective tasks and specialize.
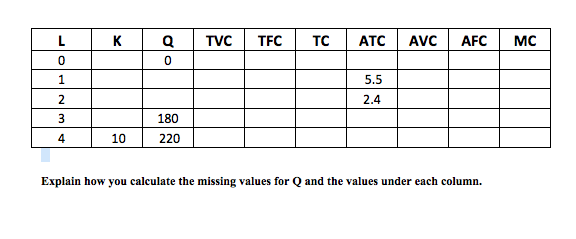
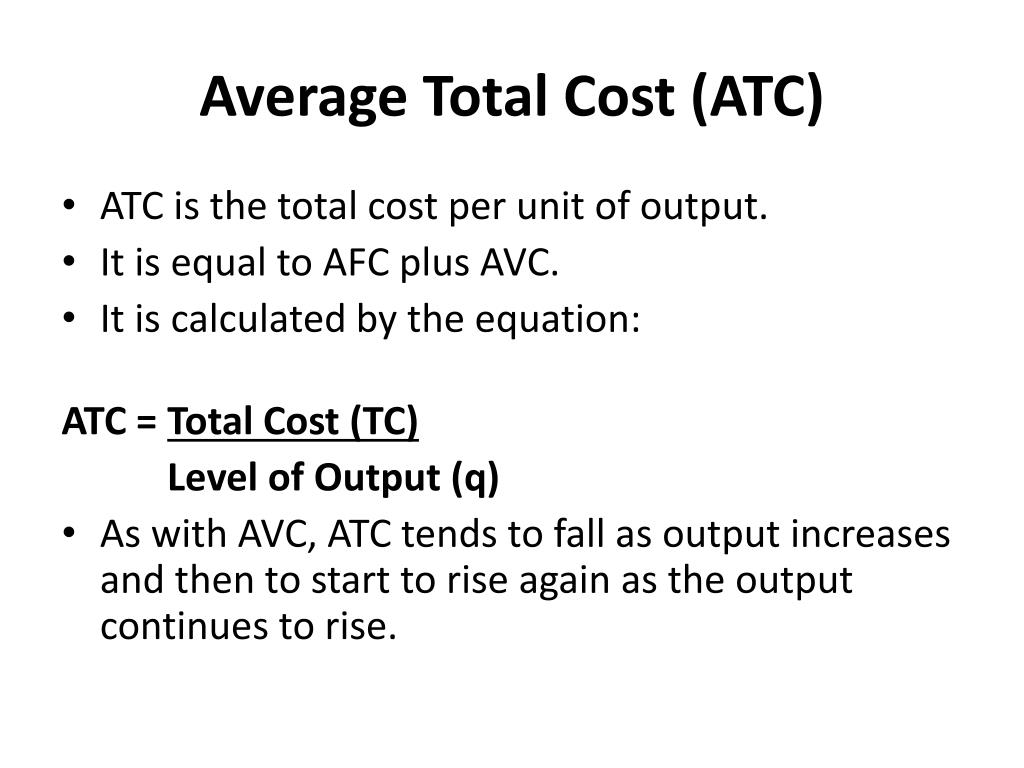
When these workers are added, the marginal product increases. With a second worker, production increases by 5 and with the third worker it increases by 6. Marginal product is the additional output that is generated by an additional worker. Total product is simply the output that is produced by all of the employed workers. Doing all of these tasks by himself, our first worker is able to produce three widgets. With one worker, the worker must fold the paper, staple it, and write the W. What will be the output level of widgets as more labor is added? With zero workers, nothing gets produced. Each round is a certain amount of time, say 40 seconds. The inputs are a stack of quarter sheets of paper, one stapler, one pen, and a 2’ x 3’ sheet of poster board which represents your factory wherein all production must take place. If you have a big family, you can do this as a Family Home Evening activity otherwise you can just read along to see the results. Our widget will be made taking a quarter sheet of paper, folding it in half twice then stapling it and writing the letter W on it. For demonstration purposes in economics, we often make widgets, which is really any hypothetical manufactured device. We can demonstrate the impact of adding more of a variable resource, say labor, to a fixed amount of capital and see what happens to output. What happens to output as more resources are employed? In order to produce, we must employ resources, i.e., land, labor, capital, and entrepreneurship. This section focuses on the second part of the equation, costs. Total revenue is equal to price times quantity and we examined their relationship in the elasticity section. Profits equal total revenue minus total costs. Have you learned all there is to know about the cost per unit curves? Check your knowledge with the worksheets below:ġ) Complete the table below by calculating the missing data: QuantityĢ) Graph the per unit (last three columns) cost curves for the table above.ģ) Explain what would happen to the per unit cost tables if total fixed cost went up by $50.Ĥ) Explain what would happen if variable cost went up by $30 at every level of production starting with unit 1.ĥ) Graph the average cost and average variable cost curves from numbers 3 and 4 above.We are now going to focus on the what is behind the supply curve. Both methods are displayed below, notice the end result is exactly the same! (Formula ATC = AFC + AVC) ) OR (Formula ATC = TC/Q). There are two methods to obtain the Average Total Cost, the first one simple takes the AFC and AVC added together, the second one takes the Total Cost figures and divides by the quantity as we did before for the AFC and AVC. Now that we analyzed Average Fixed Cost and Average Variable Cost we combine them to build the Average Total Cost, the combination of total fixed and variable, just like we did with the Total Fixed and Total Variable Costs. Below is the table for the Total Variable Cost with an added column to reflect the Average Variable cost: Remember no costs at Zero units, so the Average Variable Cost curve starts at one unit and continues at two units, three units, etc.
Average Variable Costs start high, go down and after added production go back up, almost like a smile! As production starts the work is not very efficient, after a while workers get better at their jobs and costs go down, eventually as production increases the costs of production increase, hence the shape of the curve. The next cost curve is the Average Variable Cost, again as the name implies these data is obtained by taking the Total Variable Cost and dividing by the number of units. The formula takes the Total Fixed Cost and divides by the number of units, (Formula AFC = TFC/Q) – note that there is no AFC when you produce zero units, hence the first coordinate is at 1 unit! Below is a table and a graph of the AFC: Since we start with the total fixed cost and spread this number amongst an increasing number of units, the AFC becomes smaller and smaller. As the name implies it is calculated by using the total fixed cost discussed previously and determining the average cost per unit. The Average Fixed Cost is the first cost per unit curve that we shall analyze. This section discusses the cost per unit function, how much does every unit cost?


 0 kommentar(er)
0 kommentar(er)
